- Author:
- Chris Adcock
- Subject:
- Algebra
- Material Type:
- Lesson Plan
- Level:
- Middle School
- Grade:
- 6
- Provider:
- Pearson
- Tags:
- License:
- Creative Commons Attribution Non-Commercial
- Language:
- English
- Media Formats:
- Text/HTML
Foghorns
Mouse and Elephant Heartbeats
Paper Clips
To the Moon Estimates
Wildflowers
Gallery Problems Exercise
Overview
Gallery Overview
Allow students who have a clear understanding of the content thus far in the unit to work on Gallery problems of their choosing. You can then use this time to provide additional help to students who need review of the unit's concepts or to assist students who may have fallen behind on work.
Gallery Descriptions
- Create Your Own Rate
Students create their own rate problems, given three quantities that must all be used in the problems or the answers. - Paper Clip Challenge
Students think about rate in the context of setting a record for making a paperclip chain. - The Speed of Light
Students must determine the speed of light so they can figure out how long it will take a light beam from Earth to reach the Moon (assuming it would make it there). They conduct research and perform calculations. - Tire Weight
Students connect area and a rate they may not be familiar with, tire pressure, to indirectly weigh a car. They find and add areas and do a simple rate calculation. Please note this problem requires adult supervision for the process of measuring the car tires. If no adult supervision is available, you can provide students with measurements to work with inside the classroom. Do not allow students to work with a car without permission from the owner and adult supervision. - Planting Wildflowers
Students apply area and length concepts (square miles, acres, and feet) to rectangles, choose and carry out appropriate area conversions, and show each step of their solutions. While specific solution paths will vary, all students who show good conceptualization will make at least one area conversion and show understanding about area even when dimensions and units change. This task allows several different correct solution paths. - Train Track
Students use information about laying railroad ties for the Union Pacific Railroad. These rates are different from those used elsewhere in the unit, asking how many rails per gang of workers, how long it takes to lay one mile of track, and how many spikes are needed for a mile of track. - Heartbeats
Students will investigate and compare the heartbeats of different animals and their own heartbeat. - Foghorn
Students use the relationships among seconds, minutes, and hours to find equivalent rates. Each step requires students to express an equivalent rate in terms of these different units of time. In any strong response, students use conversion factors and the given rate to find equivalent rates.
Create Your Own Rate
Answers
Answers will vary. Possible answers:
The unit price of tomatoes is $0.80 per pound. How much money do I need to buy 40 pounds of tomatoes?
I bought 40 pounds of clay for $32.00. What was the unit price per pound of clay?
I have $32.00 to buy onions that cost $0.80 per pound. How many pounds of onions can I buy?
Answers will vary. Have students check each other's problems.
- The set of quantities for problem (d) (2.25 miles, 1.8 miles, and 4.05 miles) does not describe a rate because the units are all miles. If the quantities were associated by rate, one of the terms would need to be of the form A per B.
Work Time
Create Your Own Rate
- Create a problem using two of these quantities. The answer must be the third quantity.
- 40 pounds
- $0.80 per pound
- $32.00
- Work individually to write new problems using each set of three quantities. Use the quantities to create realistic problems. Do not include the answer when you write your problems.
- 40 bags, $32.00, $0.80 per bag
- 0.15 gallon, 10.5 miles, 70 miles per gallon
- 25 feet per second, 0.4 second, 10 feet
- 2.25 miles, 1.8 miles, 4.05 miles
- 3.4 minutes, 0.4 minute per gallon, 8.5 gallons
- Do any of the sets of numbers not describe a rate? If not, explain why.
Paper Clip Challenge
Answers
Part 1
The rate would be about 0.63 paper clip per second.
Use the inverse of the rate of the number of paper clips per second.
The rate would be about 1.6 seconds per paper clip.
Answers will vary, but the rate of is easier to visualize because the number is greater than 1.
Part 2
Possible answer: If students chain 30 paper clips in 60 seconds, their rate would be 30 paper clips per 60 seconds, or 0.5 paper clip per second, which is realistically 1 paper clip every 2 seconds.
There would be paper clips in the chain after hours.Possible answer: If students chain 48 paper clips in 120 seconds, their rate would be 48 paper clips per 120 seconds, or 0.4 paper clip per second. Their rate would not have remained the same, because 0.4 paper clip per second is not the same as 0.5 paper clip per second.
There would be 34,560 paper clips in the chain after 24 hours.
Work Time
Paper Clip Challenge: Part 1
Do you have what it takes to set a record? In 2004, a record was set for chaining 54,030 paper clips in 24 hours! Watch this video to see the 2004 record.
- Express the rate in paper clips per second.
- Express the rate in seconds per paper clip.
- Which unit is more useful? Explain your choice.
VIDEO: Paper Clips
Paper Clip Challenge: Part 2
See what you can do.
- Chain together paper clips for 60 seconds.
- Count the number of paper clips in your chain. What is your rate expressed in paper clips per second?
- If you could maintain that speed for 24 hours, how many paper clips would be in your chain after 24 hours?
2. Chain together paper clips for 120 seconds.
- Count the paper clips in your chain.
- What is your rate?
- Did your rate expressed in paper clips per second change, or did it remain the same?
The Speed of Light
Answers
Carlos' estimate is closer, but it is too fast.
Answers will vary—this is a prediction.
You need to know the distance from Earth to the moon and the speed of light.
It takes a little more than seconds for light to travel from the Earth to the moon.
The calculation will vary compared to the video due to differences in rounded values.
The distance from Earth to Pluto varies: from about 4.3 billion kilometers to 7.5 billion kilometers. Time for light to travel to Pluto (when it is the nearest):
It takes about 4 hours for light to travel from Earth to Pluto when Pluto is at its nearest point to Earth.
Time for light to travel to Pluto (when it is the farthest):
It takes about 7 hours for light to travel from Earth to Pluto when Pluto is the farthest from Earth.
- Answers will vary.
- Answers will vary.
A beam of light from Earth will travel approximately 777,600,000,000 kilometers in 30 days.
This distance is more than 100 times farther away from Earth than Pluto.
Work Time
The Speed of Light
- Watch the first video. Whose estimate of the time it takes light to reach the moon do you think is closer to reality, Carlos’s or Emma’s?
- Make your own estimate of how long you think it takes light to get from Earth to the moon. Demonstrate your estimate to your partner by moving your finger across the screen.
- What information do you need to have in order to determine how long it takes light to get from Earth to the moon?
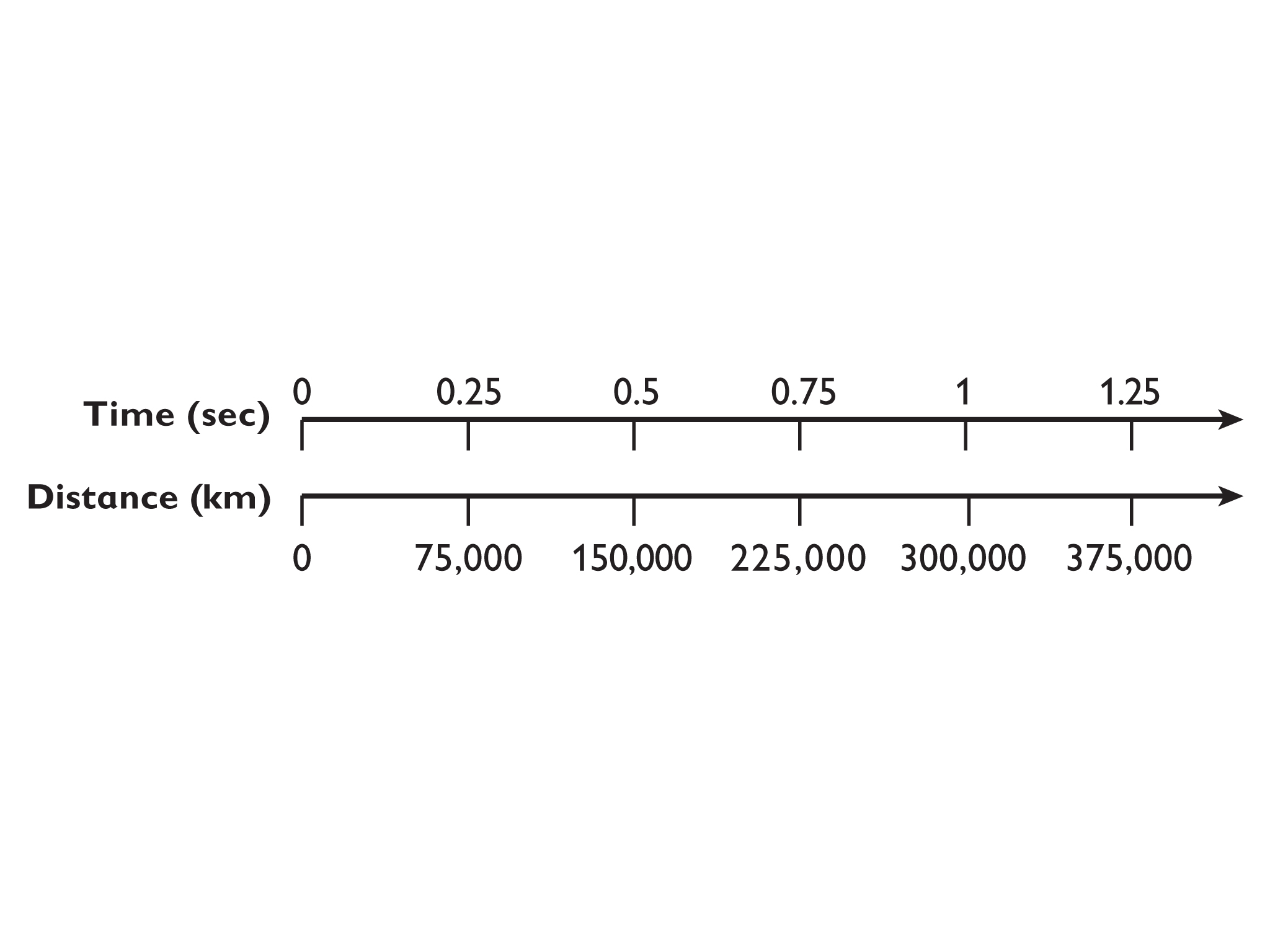
- Create a double number line to show how far light travels each second. The speed of light is approximately .
- The moon is approximately 384,400 km from Earth. How many seconds does it take light from Earth to reach the moon? Calculate your answer, and then watch the second video to check your answer.
- Why might your calculation be a little different from the actual travel time to the moon?
- Pluto is 7.5 billion km away at its farthest from Earth and billion 4.3 km away at its closest.
If the beam of a flashlight were bright enough to be seen from outer space, how long would it take a beam of light from Earth to reach Pluto when it’s farthest? When it’s closest?
- Choose an object in the universe and determine how long it would take the light from Carlos’s flashlight on Earth to reach it.
- If the light from Earth (the flashlight) takes 30 days to reach a certain point in space, how far away is that point?
VIDEO: To the Moon Estimates
VIDEO: Actual Travel Time
Tire Weight
Answers
Part 1
- Make sure students are supervised while taking tire measurements.
- Answers will vary. Possible answer: 5 in • 6 in
- Tire pressure = 35 psi
- 5 in • 6 in = 30 in2
- 30 in2 • 35 = 1,050 lbs
The tires all had the same surface area in contact with the ground.
Part 2
- Weight of the car is: 1,050 lb • 4 = 4,200 lb
- Answers will vary.
Work Time
Tire Weight: Part 1
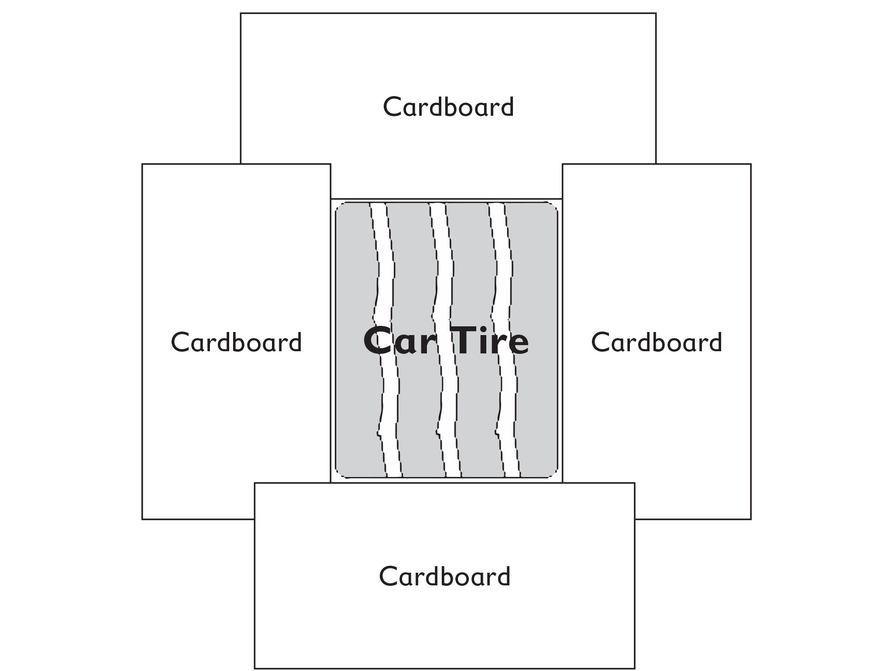
By measuring the amount of surface area each tire presses into the ground and the pressure inside each tire, you can calculate the weight of a car.
You need to measure the surface area of the bottom of each tire. Make sure you get permission from the car’s owner before you take any measurements, and take measurements only when there is an adult supervising you.
- Most tire “footprints” will be roughly rectangular in shape. To find the length and the width of this footprint, use a piece of cardboard, a piece of string, or a yardstick to measure each tire’s footprint boundary. Measure the two sides that are easiest for you to reach.
- Record your measurements in inches.
- Ask an adult to use a tire gauge to measure the internal air pressure of the tire, or use the average tire pressure of most tires: 35.
- Find the amount of tire surface area touching the ground. To find this, multiply the length and width of the footprint. Your answer should be in square inches.
To find the amount of weight the tire is holding, multiply the surface area by the pressure (in PSI) of that tire. For example:
Repeat these steps for each of the other three tires if you have time. If you do not have time, use the same measurement you took for the first tire to represent all 4 tires.
Tire Weight: Part 2
- Add the weights together for all four tires to get the total weight of the car.
- To see how close you came to the real weight of the car, ask an adult to check the owner’s manual or look at the specification plate on the inside of the driver’s side door.
If you do not have access to an owners manual here are some sample weights of typical cars:
- A small car weighs about 3,000 lb.
- A medium car weighs about 4,000 lb.
- A small truck or SUV weighs about 4,500 lb.
- A large truck or SUV weighs about 5,500 lb.
Planting Wildflowers
Answers
- You need to know the area of the median strip per mile of highway. You also need to know how many pounds of seeds are required per unit of area.
- The highway workers need 800 lbs of wildflower for every mile of highway.
- 1 mi = 5,280 ft
Area of 1 mile of a 33-foot median strip = 33 ft • 5,280 ft = 174,240 sq ft
How many square feet per acre? 43,560 sq ft = 1 acre
The highway workers need 800 lbs of wildflower seeds to plant each mile of a highway median strip that is 33 ft wide.
Work Time
Planting Wildflowers
Highway workers are going to plant wildflowers on the median strip of a length of highway. Your job is to figure out how many pounds of wildflower seeds are needed for each mile of this highway. Watch the video in order to understand the situation.
- What do you need to know to answer this problem?
Solve the problem using the following information:
- 200 pounds of seeds are needed to plant 1 acre of wildflowers.
- The median strip is 33 feet wide.
- 1 mile is 5,280 feet.
- 1 acre is 43,560 square feet.
Show each step of your solution.
VIDEO: Wildflowers
Train Track
Answers
- 30 seconds per rail per gang; 4 rails per minute; 10 spikes per rail; 400 rails per mile.
- Each gang lays down 1 rail every 30 seconds, or 2 rails per minute. There must be 2 gangs working since 4 rails are being laid per minute.
- It takes 100 minutes, or 1 hour and 40 minutes, for 2 gangs to lay down 1 mile of railroad track.
- There are 4,000 spikes needed for 1 mile of railroad track.
Work Time
Train Track
Read this quote about the Union Pacific Railroad from 1867.
“Thirty seconds to a rail for each gang, so four rails go down a minute. There are ten spikes to a rail, 400 rails to a mile.”
A gang is a work crew who lays down the track for the train. The track consists of rails and spikes that hold the rails in place.
- List the different rates described in the quote.
- How many gangs are at work in the scene described in the quotation? Show your work.
- How long does it take 2 gangs to lay down 1 mile of railroad track? Show your work.
- How many spikes are needed to lay down 1 mile of railroad track? Show your work.
Heartbeats
Answers
- Predictions will vary.
- Predictions will vary.
- Resting heart rates will vary.
- Possible answer: Each line of the double number line represents an axis of the graph. The vertical axis on the graph and the top line of the double number line both show the number of beats. The horizontal axis on the graph and the bottom line of the double number line both show the time in seconds. A point on the graph represents the combination of two corresponding numbers on the double number line. Both the graph and the double number line show a constant rate: the points on the graph form a regular, linear pattern, while the points on the double number line appear at equally spaced intervals.
- Possible answer: The point at (10,12) means that in 10 seconds there were 12 beats.
- Answers will vary. Students should note that the graphs of higher heart rates will have steeper lines than the graphs of lower heart rates.
- Answers will vary. In general, small animals have more rapid heartbeats.
Work Time
Heartbeats
- Predict what you would hear if you listened to the heartbeats of an elephant and a mouse. Follow the instructions in the interactive to hear the heartbeats of the elephant and the mouse.
- Predict what your heartbeat would sound like compared with the mouse’s and the elephant’s heartbeats. Do you think your heartbeat is faster or slower?
- Find your pulse. Follow the instructions in the Heartbeats interactive to record your pulse and heart rate.
- Compare the graph with the double number line. How are they alike? How are they different?
- Pick a point on the graph. Explain what it means.
- How does your heart rate compare with a mouse’s heart rate? How does it compare with an elephant’s heart rate?
- Choose an animal and research its heart rate. Predict what the graph of its heart rate would look like. How would the graph compare with your heart rate graph?
INTERACTIVE: Mouse and Elephant Heartbeats
Foghorn
Answers
The rate is blasts per minute.
The foghorn will blast times in hour.
The lighthouse keeper fired the cannon at a rate of about 0.3 blast per minute, or realistically 1 blast every 3 minutes.
The Beavertail Lighthouse foghorn is checked about every hours, or about every days.
If the cannon was also checked after the same number of blasts, it would be checked every 500 hours, or about every 21 days.
Answers will vary.
Work Time
Foghorn
A foghorn is a device that uses sound to warn vehicles of hazards in foggy conditions. Many lighthouses have foghorns that blast at periodic intervals. Watch the video to learn about foghorns.
The Beavertail Lighthouse in Rhode Island blasts its foghorn once every 30 seconds.
- What is the rate expressed in blasts per minute?
- How many times will the foghorn blast in 1 hour?
Before electronic foghorns were invented, the lighthouse keeper periodically fired a cannon. If the lighthouse keeper fired the cannon 20 times per hour, what is the rate in blasts per minute?
Foghorns are checked after 10,000 blasts.
- How often is the Beavertail Lighthouse foghorn checked?
- If the cannon was also checked at the same rate, how often would that be?
Research the foghorns at the Golden Gate Bridge in San Francisco. The foghorns are located in two locations: at the middle of the bridge (midspan) and at the south (San Francisco) tower pier. The foghorns have different sounds. Create a problem about their rates.
VIDEO: Foghorns